题目大意：

给你r1，r2，表示两个无限长的圆柱体的半径，然后这两个圆柱体的中心线相互垂直，求重复的体积。

解题思路：

这题一开始准备直接暴力积分做，然后滚粗了，不是挂精度就是wa了，所以后来就膜拜大神的做法去了，大神用的是自适应辛普森，当这个函数的(a,b)段接近二次函数的时候，这一段的积分就是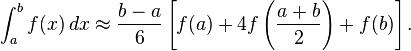，然后我想这道题不就是近似二次函数吗(也可能是我搞错了)，然后就直接对(0,MIN( r1 ,r2 ))用了这个公式，然后滚粗了，后来问了一个大神，他说需要有一个判断条件(假设G(l，r)代表的就是上述式子) 必须有fabs(G(l，r)-G(l，(l+r)/2)-G((l+r)/2，r))<=EPS(EPS请自己定义)，这个式子公式才比较准确。然后用了一下，果然AC了。

AC代码：

#include <cstdio>
#include <cmath>
#include <cstdlib>
#include <cstring>
#include <iostream>
#include <algorithm>
#define MIN(a,b) ((a)>(b)?(b):(a))
#define G(a,b) ((F(a)+F(b)+F((a+b)/2)*4)*(b-a)/6)

using namespace std;
double r1,r2;
double RM;
const double EPS=1e-7;
double ans=0;

inline double F(double x)
{return sqrt((r1*r1-x*x)*(r2*r2-x*x));}

inline void Count(double l,double r)
{
	if(fabs(G(l,r)-G(l,(l+r)/2)-G((l+r)/2,r))<=EPS)
		ans+=G(l,r)*8;
	else
	{
		Count(l,(l+r)/2);
		Count((l+r)/2,r);
	}
	return;
}

int main()
{
	cin>>r1>>r2;
	RM=MIN(r1,r2);
	
	Count(0,RM);
	
	printf("%lf\n",ans);
	return 0;
}

sgu-217 Two Cylinders